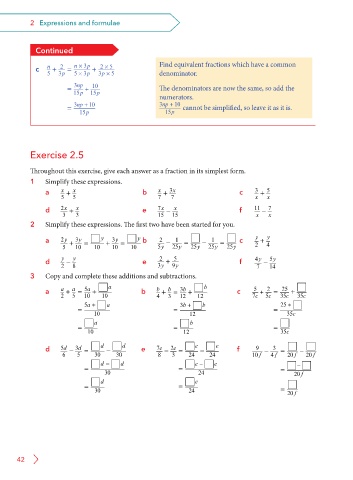
Page 43 - 新思维数学学生用书9 样章

2 Expressions and formulae

Continued

c n + 2 = n × 3 p + 2 × 5 Find equivalent fractions which have a common
5 3 p 5 × 3 p 3 p × 5 denominator.

= 3 np + 10 The denominators are now the same, so add the
15 p 15 p
numerators.
= 3 np + 10 3np + 10 cannot be simplified, so leave it as it is.
15 p 15p

Exercise 2.5

Throughout this exercise, give each answer as a fraction in its simplest form.
1 Simplify these expressions.
a x + x b x + 3 x c 3 + 5
5 5 7 7 x x

d 2x + x e 7x − x f 11 − 7
3 3 15 15 x x
2 Simplify these expressions. The first two have been started for you.

a 2y + 3y = y + 3y = y b 2 − 1 = − 1 = c y + y
5 10 10 10 10 5y 25y 25y 25y 25y 2 4
d y − y e 2 + 5 f 4y − 5y
2 8 3y 9y 7 14
3 Copy and complete these additions and subtractions.

a a + a = 5 a + a b b + b = 3 b + b c 5 + 2 = 25 +
2 5 10 10 4 3 12 12 7c 5c 35c 35c
5 a + a 3 b + b 25 +
= = =
10 12 35c
a b
= = =
10 12 35c

d 5d − 3d = d − d e 7e − 2e = e − e f 9 − 3 = −
6 5 30 30 8 3 24 24 10 f 4 f 20 f 20 f
d − d e − e −
= = =
30 24 20 f
d e
= =
30 24 =
20 f

42