Page 14 - 新思维数学活动用书9 样章

2.1 Substituting into expressions

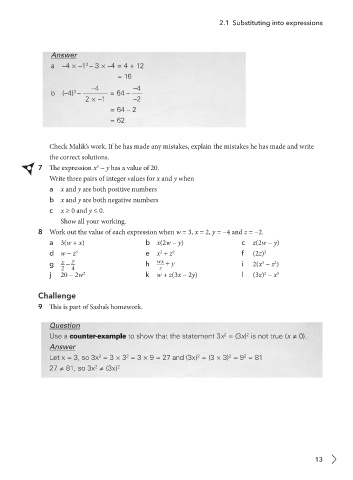
Answer
a –4 × –1 – 3 × –4 = 4 + 12
2
= 16
–4 –4
b (–4) – = 64 –
3
2 × –1 –2
= 64 – 2
= 62

Check Malik’s work. If he has made any mistakes, explain the mistakes he has made and write

the correct solutions.
7 The expression x − y has a value of 20.
3
Write three pairs of integer values for x and y when
a x and y are both positive numbers

b x and y are both negative numbers
c x ≥ 0 and y ≤ 0.
Show all your working.
8 Work out the value of each expression when w = 3, x = 2, y = −4 and z = −2.

a 3(w + x) b x(2w − y) c z(2w − y)
d w − z 3 e x + z 2 f (2z) 3
2
g x − y h wx + y i 2(x − z )
2
3
z
2
4
j 20 − 2w 2 k w + z(3x − 2y) l (3z) − x 5
2
Challenge
9 This is part of Sasha’s homework.

Question
Use a counter-example to show that the statement 3x = (3x) is not true (x ≠ 0).
2
2
Answer
Let x = 3, so 3x = 3 × 3 = 3 × 9 = 27 and (3x) = (3 × 3) = 9 = 81
2
2
2
2
2
27 ≠ 81, so 3x ≠ (3x) 2
2

13